or, how to get a round cake into a square tin.

Every year about this time I think about making the Christmas cake and find lots of recipes for circular Christmas cakes. But Christmas cakes, in my eyes, must be square! This is probably mostly because my mum made square cakes in my childhood but also because square cakes are much easier to cut into slices of the exact size you feel like eating. So every year I find myself wondering how to convert a recipe for a round tin to fit in a square tin.

So every year I work out the area of an 8 inch circular tin and see that I need to put the same amount of cake batter into a 7 inch square tin. Or I work out the area of a 9 inch circular tin and see that I can make the same cake in an 8 inch square tin.

I see the inklings of a rule of thumb “A cake meant for an n inch round tin can be made in an n-1 inch square tin” - but how useful is that?

The formula for the area of a circle is πr2. Since cake tins are usually measured by their diameters rather than their radii the radius I want to plug into the formula is n/2. The formula for the area of a square, with sides an inch smaller than the circle n-1, is simply (n-1)2.

I only care about the area of the round/square tin, not its volume, as I generally want the cake to be the same thickness whatever shape it is. What I want to know how close together these areas are to know whether our rule of thumb holds across different size cake tins.

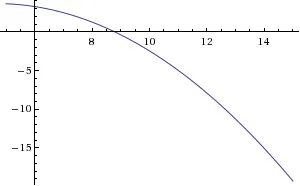
How I do this is to take the area of the round tin then take away the area of the square tin. This tells me how many more square inches are in the round tin than in the square one. This function is:  π(n/2)2 - (n-1)2.

I like graphs so I plot this function. Wolfram Alpha is great at this. (Cue wavy line story about the old days when we had to write graphing code with our bare hands, uphill both ways, with only a 80386 processor between us.)

I ask Wolfram Alpha to plot “plot pi*(n/2)^2 - (n-1)^2 between n=5 and n=15” for me. The limits are there because a cake tin smaller than 5 inches diameter or larger than 15 inches diameter is not something a domestic cook ever needs to worry about!

So it looks like the rule of thumb holds up pretty well between about 7 and 11 inch round tins, below about 9 inches we have a bit too much batter to fill a square tin of an inch smaller size, and above that we have not quite enough. From 11 inches on it looks very much like we have a lot less batter than required and will end up with a thin cake. But I haven’t accounted for the fact that there will be more batter anyway in a bigger cake. I ought to look at the ratio (or percentage) of how much less batter we have to what we need rather than just how much less we have. To do this we divide the extra area from the above formula by the area of the square tin. In Wolfram Alpha speak that is “plot (pi*(n/2)^2 - (n-1)^2)/((n-1)^2) between n=5 and n=15”.

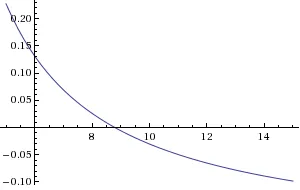
This shows that moving a recipe for a 6 inch round cake to a 5 inch square tin will give you about 12% more cake batter than you need. At the other end moving from a 15 inch round tin to a 14 inch square one gives you about 10% less batter than required so you’ll get a thinner cake.

To me this sounds well within reasonable limits and I’m happy to say that you should always make an n inch round cake recipe in an n-1 inch square tin.

The only thing that’s bothering me now is that thin cake in a big tin - when would you be better off moving to an n-2 inch tin to get a thicker cake again? Back to Wolfram Alpha again… I won’t spell out all the details as by now you will either be with me or completely lost, but it looks like at about a 14 inch round tin you could either use a 13 inch square tin and have a cake 10% thinner, or go down to a 12 inch tin and have a cake 10% thicker than the round one. 12 inch square Christmas cakes are a bit out of my league though, after all there is Christmas pudding and gingerbread houses to get through before you even get to the Twiglets so I think I’m happy with my rule of thumb!

Primary schools always tell you that maths is useful for cooking, and so it is, I think they had basic arithmetic in mind really though!

And just in case anyone reading this is actually planning on making an n inch round cake in an n-1 inch square tin my experience is that the cooking time is about the same and usually depends more on the idiosyncrasies of the oven than the mathematics of cake volume.